Chapter 3 Review Algebra 1 Answers 3-1 Through 3-3

Chapter 3 Review Exercises

1. Terminal updated

2. Save as PDF

Page ID

18494

Chapter Review Exercises

Graph Linear Equations in Two Variables

Plot Points in a Rectangular Coordinate Arrangement

In the post-obit exercises, plot each signal in a rectangular coordinate system.

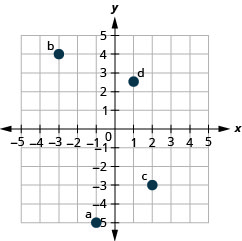
1. ⓐ \((−one,−v)\)
ⓑ \((−3,4)\)
ⓒ \((2,−3)\)
ⓓ \((1,\frac{5}{2})\)

Answer

2. ⓐ \((−two,0)\)
ⓑ \((0,−4)\)
ⓒ \((0,5)\)
ⓓ \((3,0)\)

In the following exercises, decide which ordered pairs are solutions to the given equations.

3. \(5x+y=x\);

ⓐ \((5,ane)\)
ⓑ \((two,0)\)
ⓒ \((4,−10)\)

Answer

ⓑ, ⓒ

iv. \(y=6x−2\);

ⓐ \((1,4)\)
ⓑ \((13,0)\)
ⓒ \((6,−2)\)

Graph a Linear Equation by Plotting Points

In the following exercises, graph by plotting points.

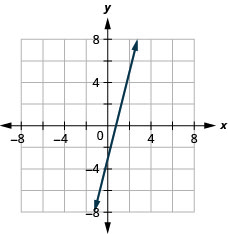
five. \(y=4x−iii\)

Answer

6. \(y=−3x\)

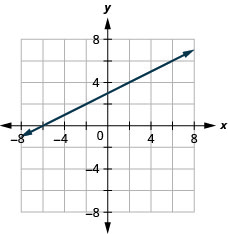
7. \(y=\frac{1}{two}x+3\)

Answer

8. \(y=−\frac{4}{five}|ten−one\)

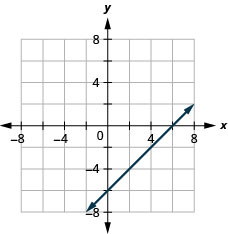
nine. \(10−y=6\)

Reply

x. \(2x+y=7\)

11. \(3x−2y=6\)

Respond

Graph Vertical and Horizontal lines

In the following exercises, graph each equation.

12. \(y=−two\)

13. \(ten=3\)

Answer

In the following exercises, graph each pair of equations in the same rectangular coordinate system.

14. \(y=−2x\) and \(y=−2\)

fifteen. \(y=\frac{4}{3}10\) and \(y=\frac{four}{3}\)

Answer

Find x- and y-Intercepts

In the following exercises, observe the 10- and y-intercepts.

16.

17.

Answer

\((0,three)(3,0)\)

In the following exercises, observe the intercepts of each equation.

18. \(x−y=−1\)

nineteen. \(x+2y=half dozen\)

Answer

\((6,0),\space (0,three)\)

20. \(2x+3y=12\)

21. \(y=\frac{3}{4}x−12\)

Answer

\((xvi,0),\space (0,−12)\)

22. \(y=3x\)

Graph a Line Using the Intercepts

In the following exercises, graph using the intercepts.

23. \(−x+3y=3\)

Answer

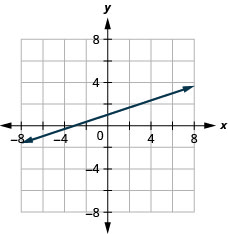
24. \(10−y=4\)

25. \(2x−y=v\)

Answer

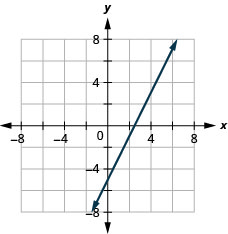
26. \(2x−4y=8\)

27. \(y=4x\)

Answer

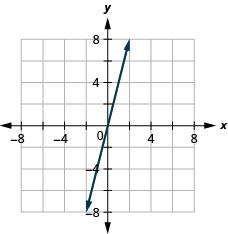
Gradient of a Line

Find the Slope of a Line

In the following exercises, find the gradient of each line shown.

28.

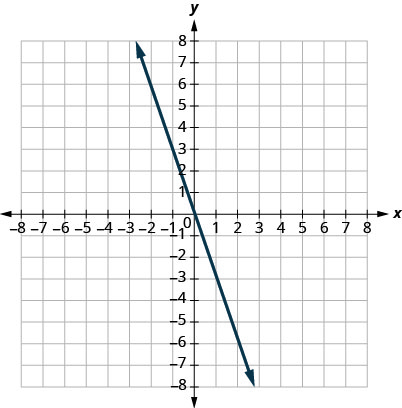
29.

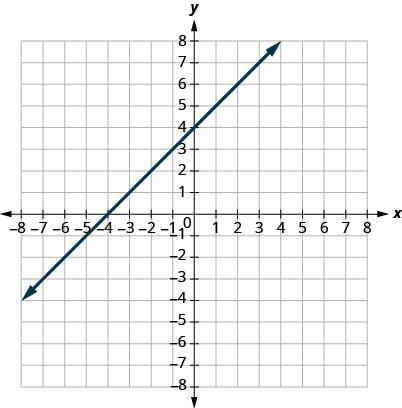
Answer

one

30.

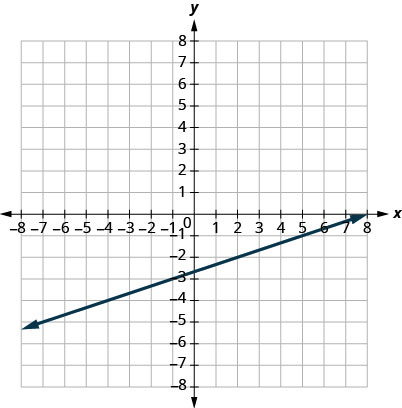
31.

Answer

\(−12\)

In the following exercises, detect the slope of each line.

32. \(y=2\)

33. \(x=five\)

Answer

undefined

34. \(x=−3\)

Use the Slope Formula to find the Slope of a Line between Two Points

In the following exercises, use the slope formula to find the slope of the line between each pair of points.

36. \((−1,−i),(0,five)\)

37. \((three.5),(4,−1)\)

Answer

\(−half dozen\)

38. \((−5,−2),(three,ii)\)

39. \((2,1),(iv,vi)\)

Answer

\(52\)

Graph a Line Given a Point and the Gradient

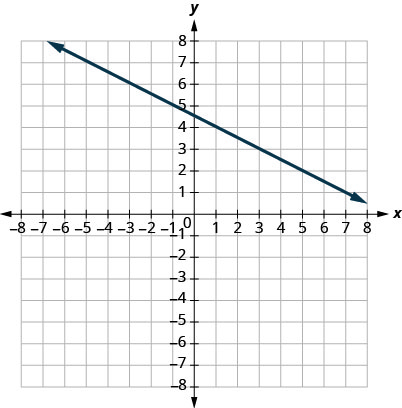
In the following exercises, graph each line with the given signal and slope.

40. \((2,−2);\space m=52\)

41. \((−3,iv);\space thousand=−13\)

Answer

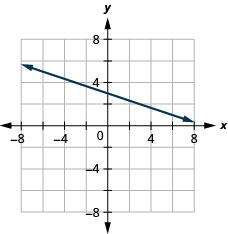
42. \(10\)-intercept \(−4; m=3\)

43. \(y\)-intercept \(i; m=−34\)

Answer

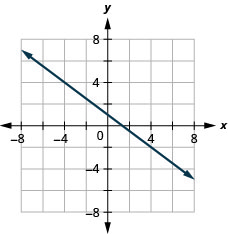
Graph a Line Using Its Slope and Intercept

In the following exercises, identify the slope and \(y\)-intercept of each line.

44. \(y=−4x+9\)

45. \(y=53x−vi\)

Answer

\(m=53;\space (0,−6)\)

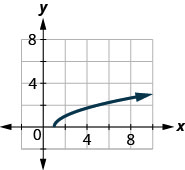
46. \(5x+y=10\)

47. \(4x−5y=8\)

Respond

\(thousand=45;\space (0,−85)\)

In the following exercises, graph the line of each equation using its slope and y-intercept.

48. \(y=2x+3\)

49. \(y=−x−1\)

Answer

50. \(y=−25x+3\)

51. \(4x−3y=12\)

Respond

In the following exercises, determine the most convenient method to graph each line.

52. \(x=5\)

53. \(y=−3\)

Answer

horizontal line

54. \(2x+y=five\)

55. \(ten−y=2\)

Answer

intercepts

56. \(y=22x+two\)

57. \(y=34x−i\)

Reply

plotting points

Graph and Interpret Applications of Slope-Intercept

58. Katherine is a individual chef. The equation \(C=6.5m+42\) models the relation betwixt her weekly cost, C, in dollars and the number of meals, one thousand, that she serves.

ⓐ Observe Katherine'southward cost for a calendar week when she serves no meals.
ⓑ Find the cost for a week when she serves xiv meals.
ⓒ Translate the gradient and C-intercept of the equation.
ⓓ Graph the equation.

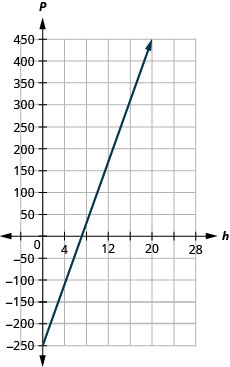
59. Marjorie teaches pianoforte. The equation \(P=35h−250\) models the relation between her weekly profit, P, in dollars and the number of student lessons, south, that she teaches.

ⓐ Find Marjorie'due south profit for a week when she teaches no student lessons.
ⓑ Find the profit for a calendar week when she teaches xx educatee lessons.
ⓒ Interpret the slope and P-intercept of the equation.
ⓓ Graph the equation.

Answer

ⓐ \(−$250\)
ⓑ \($450\)
ⓒ The slope, 35, means that Marjorie's weekly profit, P, increases by $35 for each additional student lesson she teaches.
The P-intercept ways that when the number of lessons is 0, Marjorie loses $250.
ⓓ

Use Slopes to Identify Parallel and Perpendicular Lines

In the following exercises, employ slopes and \(y\)-intercepts to determine if the lines are parallel, perpendicular, or neither.

60. \(4x−3y=−1;\quad y=43x−3\)

61. \(y=5x−ane;\quad 10x+2y=0\)

Answer

neither

62. \(3x−2y=five;\quad 2x+3y=six\)

63. \(2x−y=viii;\quad x−2y=iv\)

Answer

not parallel

Find the Equation of a Line

Find an Equation of the Line Given the Gradient and y-Intercept

In the post-obit exercises, detect the equation of a line with given gradient and y-intercept. Write the equation in slope–intercept form.

64. Slope \(\frac{1}{3}\) and \(y\)-intercept \((0,−6)\)

65. Slope \(−5\) and \(y\)-intercept \((0,−3)\)

Answer

\(y=−5x−3\)

66. Gradient \(0\) and \(y\)-intercept \((0,4)\)

67. Slope \(−2\) and \(y\)-intercept \((0,0)\)

Answer

\(y=−2x\)

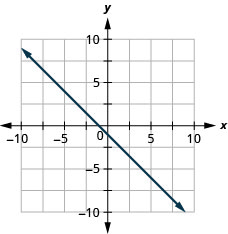
In the following exercises, find the equation of the line shown in each graph. Write the equation in slope–intercept form.

68.

69.

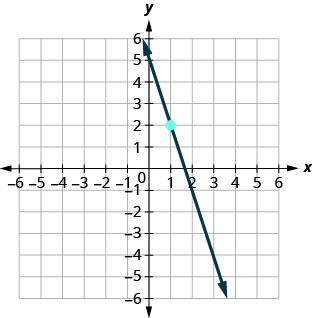
Answer

\(y=−3x+5\)

seventy.

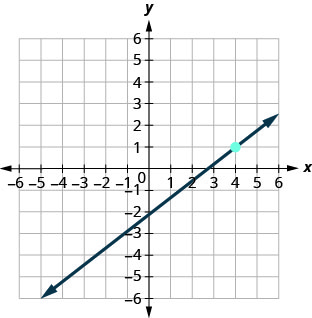
71.

Answer

\(y=−4\)

Observe an Equation of the Line Given the Slope and a Bespeak

In the post-obit exercises, find the equation of a line with given slope and containing the given point. Write the equation in slope–intercept form.

72. \(grand=−\frac{1}{4}\), point \((−8,3)\)

73. \(grand=\frac{3}{5}\), point \((10,6)\)

Answer

\(y=\frac{three}{v}x\)

74. Horizontal line containing \((−2,seven)\)

75. \(m=−2\), point \((−i,−three)\)

Answer

\(y=−2x−v\)

Find an Equation of the Line Given Two Points

In the post-obit exercises, notice the equation of a line containing the given points. Write the equation in slope–intercept form.

76. \((2,10)\) and \((−2,−2)\)

77. \((7,ane)\) and \((5,0)\)

Reply

\(y=\frac{i}{2}x−\frac{5}{2}\)

78. \((3,viii)\) and \((three,−4)\)

79. \((v,2)\) and \((−1,2)\)

Answer

\(y=2\)

Discover an Equation of a Line Parallel to a Given Line

In the following exercises, observe an equation of a line parallel to the given line and contains the given indicate. Write the equation in slope–intercept course.

80. line \(y=−3x+six\), betoken \((1,−5)\)

81. line \(2x+5y=−10\), indicate \((10,4)\)

Respond

\(y=−\frac{2}{5}x+8\)

82. line \(x=4\), point \((−2,−1)\)

83. line \(y=−five\), point \((−four,3)\)

Answer

\(y=3\)

Find an Equation of a Line Perpendicular to a Given Line

In the following exercises, find an equation of a line perpendicular to the given line and contains the given signal. Write the equation in slope–intercept form.

84. line \(y=−\frac{four}{5}ten+2\), indicate \((8,9)\)

85. line \(2x−3y=nine\), point \((−4,0)\)

Answer

\(y=−\frac{3}{2}x−6\)

86. line \(y=3\), point \((−1,−3)\)

87. line \(10=−v\) point \((ii,1)\)

Answer

\(y=one\)

Graph Linear Inequalities in Two Variables

Verify Solutions to an Inequality in Two Variables

In the following exercises, determine whether each ordered pair is a solution to the given inequality.

88. Determine whether each ordered pair is a solution to the inequality \(y<10−3\):

ⓐ \((0,1)\) ⓑ \((−two,−4)\) ⓒ \((five,2)\) ⓓ \((3,−1)\)
ⓔ \((−i,−v)\)

89. Determine whether each ordered pair is a solution to the inequality \(x+y>4\):

ⓐ \((half-dozen,ane)\) ⓑ \((−iii,vi)\) ⓒ \((three,ii)\) ⓓ \((−5,ten)\) ⓔ \((0,0)\)

Answer

Recognize the Relation Betwixt the Solutions of an Inequality and its Graph

In the post-obit exercises, write the inequality shown by the shaded region.

90. Write the inequality shown by the graph with the boundary line \(y=−x+2.\)

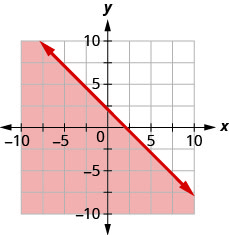
91. Write the inequality shown by the graph with the boundary line \(y=\frac{two}{3}ten−3\).

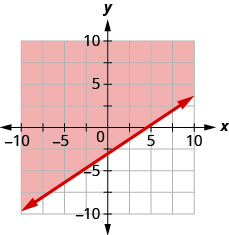
Answer

\(y>\frac{ii}{iii}ten−3\)

92. Write the inequality shown past the shaded region in the graph with the boundary line \(10+y=−4\).

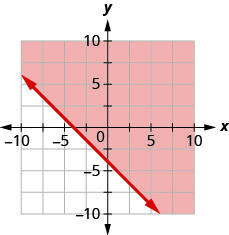
93. Write the inequality shown past the shaded region in the graph with the purlieus line \(ten−2y=six\).

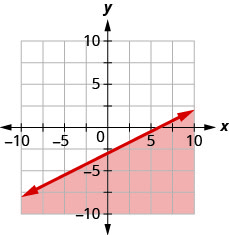
Answer

\(ten−2y\geq 6\)

Graph Linear Inequalities in Two Variables

In the following exercises, graph each linear inequality.

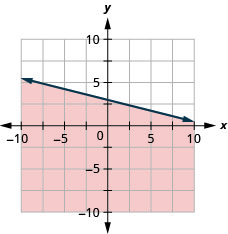
94. Graph the linear inequality \(y>\frac{2}{5}10−4\).

95. Graph the linear inequality \(y\leq −\frac{1}{iv}x+three\).

Answer

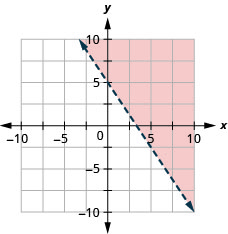
96. Graph the linear inequality \(10−y\leq 5\).

97. Graph the linear inequality \(3x+2y>10.\)

Respond

98. Graph the linear inequality \(y\leq −3x\).

99. Graph the linear inequality \(y<half dozen.\)

Answer

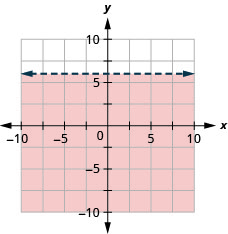
Solve Applications using Linear Inequalities in Ii Variables

100. Shanthie needs to earn at least $500 a calendar week during her summertime break to pay for college. She works two jobs. One equally a swimming teacher that pays $x an 60 minutes and the other as an intern in a police force office for $25 hour. How many hours does Shanthie demand to piece of work at each job to earn at least $500 per week?

ⓐ Let 10 exist the number of hours she works didactics pond and let y be the number of hours she works as an intern. Write an inequality that would model this situation.
ⓑ Graph the inequality.
ⓒ Find three ordered pairs \((x,y)\) that would exist solutions to the inequality. And so, explain what that means for Shanthie.

101. Atsushi he needs to exercise enough to burn \(600\) calories each day. He prefers to either run or bike and burns \(20\) calories per minute while running and \(15\) calories a infinitesimal while biking.

ⓐ If 10 is the number of minutes that Atsushi runs and y is the number minutes he bikes, find the inequality that models the situation.
ⓑ Graph the inequality.
ⓒ List three solutions to the inequality. What options do the solutions provide Atsushi?

Answer

ⓐ \(20x+15y\geq 60020x+15y\geq 600\)
ⓑ

ⓒ Answers will vary.

Relations and Functions

Detect the Domain and Range of a Relation

In the post-obit exercises, for each relation, ⓐ detect the domain of the relation ⓑ find the range of the relation.

102. \({\{(five,−2),\,(5,−iv),\,(7,−vi),\,(8,−8),\,(9,−x)}\}\)

103. \({\{(−iii,7),\,(−2,3),\,(−i,nine), \,(0,−3),\,(−one,8)}\}\)

Answer

ⓐ \(D: {−3, −ii, −1, 0}\)
ⓑ \(R: {7, 3, 9, −iii, 8}\)

In the post-obit exercise, utilise the mapping of the relation to ⓐ listing the ordered pairs of the relation ⓑ find the domain of the relation ⓒ find the range of the relation.

104. The mapping below shows the average weight of a kid according to age.

In the post-obit practice, use the graph of the relation to ⓐ list the ordered pairs of the relation ⓑ find the domain of the relation ⓒ observe the range of the relation.

105.

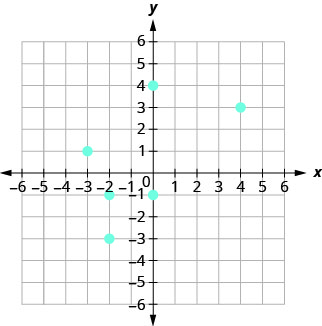
Answer

ⓐ \((iv, 3), \,(−2, −3), \,(−2, −one), \,(−3, i), \,(0, −ane), \,(0, 4)\)
ⓑ \(D: {−3, −2, 0, iv}\)
ⓒ \(R: {−three, −1, ane, 3, iv}\)

Determine if a Relation is a Function

In the following exercises, apply the set of ordered pairs to ⓐ decide whether the relation is a function ⓑ find the domain of the relation ⓒ find the range of the relation.

106. \({\{(9,−five),\,(4,−3),\,(1,−i),\,(0,0),\,(1,ane),\,(iv,3),\,(ix,v)}\}\)

107. \({\{(−3,27),\,(−2,8),\,(−1,one),\,(0,0),\,(1,1),\,(two,viii),\,(iii,27)}\}\)

Respond

ⓐ yes ⓑ \({−3, −2, −1, 0, 1, 2, 3}\)
ⓒ \({0, 1, viii, 27}\)

In the post-obit exercises, use the mapping to ⓐ determine whether the relation is a function ⓑ find the domain of the office ⓒfind the range of the function.

108.

109.

Answer

ⓐ \({−3, −2, −1, 0, 1, 2, iii}\)
ⓑ \({−three, −ii, −1, 0, 1, 2, 3}\)
ⓒ \({−243, −32, −1, 0, 1, 32, 243}\)

In the following exercises, decide whether each equation is a part.

110. \(2x+y=−3\)

111. \(y=ten^2\)

Answer

yes

112. \(y=3x−five\)

113. \(y=x^3\)

Answer

aye

114. \(2x+y2=4\)

Find the Value of a Part

In the following exercises, evaluate the function:

ⓐ \(f(−2)\) ⓑ \(f(3)\) ⓒ \(f(a)\).

115. \(f(x)=3x−four\)

Answer

ⓐ \(f(−2)=−10\) ⓑ \(f(three)=5\) ⓒ \(f(a)=3a−4\)

116. \(f(x)=−2x+5\)

117. \(f(ten)=x^2−5x+6\)

Answer

ⓐ \(f(−2)=20\) ⓑ \(f(3)=0\) ⓒ \(f(a)=a^2−5a+6\)

118. \(f(10)=3x^ii−2x+ane\)

In the following exercises, evaluate the office.

119. \(g(x)=3x2−5x;\space g(ii)\)

Reply

\(2\)

120. \(F(x)=2x2−3x+1;\infinite F(−1)\)

121. \(h(t)=iv|t−1|+2;\infinite h(t)=iv\)

Reply

\(18\)

122. \(f(x)=x+2x−one;\space f(3)\)

Graphs of Functions

Utilize the Vertical line Test

In the following exercises, decide whether each graph is the graph of a function.

123.

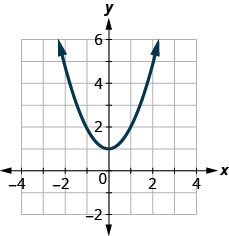
Answer

yes

124.

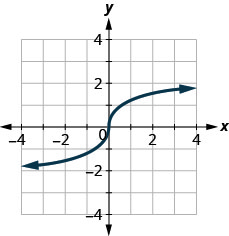
125.

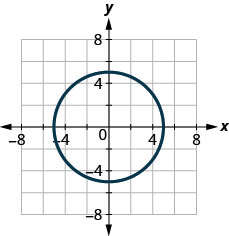
Reply

no

126.

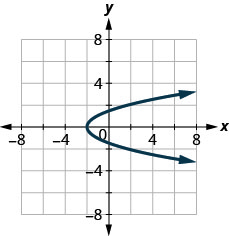
127.

Answer

yes

128.

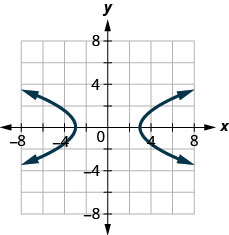
129.

Answer

no

Place Graphs of Basic Functions

In the following exercises, ⓐ graph each part ⓑ state its domain and range. Write the domain and range in interval notation.

130. \(f(x)=5x+1\)

131. \(f(10)=−4x−ii\)

Answer

ⓐ

ⓑ \(D: (-\inf ,\inf ), R: (-\inf ,\inf )\)

132. \(f(x)=\frac{2}{3}x−i\)

133. \(f(x)=−6\)

Answer

ⓐ

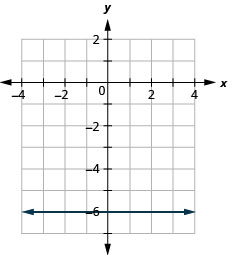
ⓑ \(D: (-\inf ,\inf ), R: (-\inf ,\inf )\)

134. \(f(x)=2x\)

135. \(f(x)=3x^2\)

Answer

ⓐ

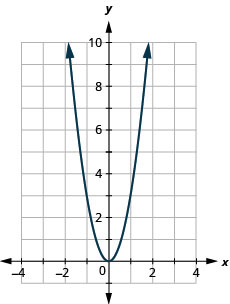
ⓑ \(D: (-\inf ,\inf ), R: (-\inf ,0]\)

136. \(f(ten)=−12x^2\)

137. \(f(10)=x^ii+ii\)

Reply

ⓐ

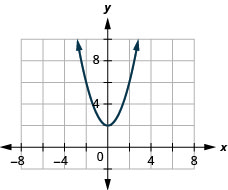
ⓑ \(D: (-\inf ,\inf ), R: (-\inf ,\inf )\)

138. \(f(ten)=10^3−ii\)

139. \(f(x)=\sqrt{x+2}\)

Answer

ⓐ

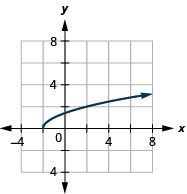
ⓑ \(D: [−2,−2, \inf ), \infinite R: [0,\inf )\)

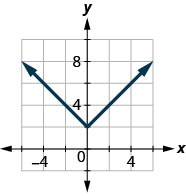
140. \(f(ten)=−|10|\)

141. \(f(x)=|10|+one\)

Answer

ⓐ

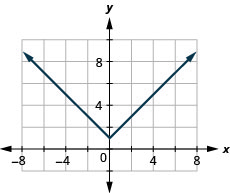
ⓑ \(D: (-\inf ,\inf ), \space R: [1,\inf )\)

Read Information from a Graph of a Function

In the following exercises, utilize the graph of the role to discover its domain and range. Write the domain and range in interval annotation

142.

143.

Answer

\(D: (-\inf ,\inf ), R: [2,\inf )\)

144.

In the post-obit exercises, use the graph of the office to find the indicated values.

145.

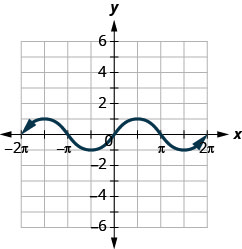
ⓐ Find \(f(0)\).
ⓑ Find \(f(12\pi )\).
ⓒ Find \(f(−32\pi )\).
ⓓ Find the values for \(x\) when \(f(x)=0\).
ⓔ Detect the \(x\)-intercepts.
ⓕ Observe the \(y\)-intercept(south).
ⓖ Find the domain. Write information technology in interval annotation.
ⓗ Find the range. Write it in interval annotation.

Answer

ⓐ \(f(x)=0\) ⓑ \(f(\pi /2)=one\)
ⓒ \(f(−three\pi /2)=1\) ⓓ \(f(x)=0\) for \(x=−2\pi ,−\pi ,0,\pi ,2\pi\)
ⓔ \((−two\pi ,0), (−\pi ,0), (0,0), (\pi ,0), (2\pi ,0)\) ⓕ \((0,0)\)
ⓖ \([−ii\pi ,2\pi ]\) ⓗ \([−1,ane]\)

146.

ⓐ Discover \(f(0)\).
ⓑ Find the values for \(x\) when \(f(10)=0\).
ⓒ Find the \(x\)-intercepts.
ⓓ Notice the \(y\)-intercept(southward).
ⓔ Find the domain. Write information technology in interval note.
ⓕ Notice the range. Write it in interval notation.

Practice Exam

1. Plot each point in a rectangular coordinate organisation.

ⓐ \((two,five)\)
ⓑ \((−i,−3)\)
ⓒ \((0,ii)\)
ⓓ \((−iv,32)\)
ⓔ \((5,0)\)

Answer

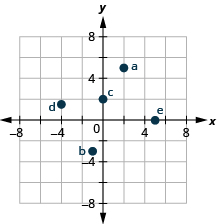
2. Which of the given ordered pairs are solutions to the equation \(3x−y=6\)?

ⓐ \((3,3)\) ⓑ \((2,0)\) ⓒ \((four,−vi)\)

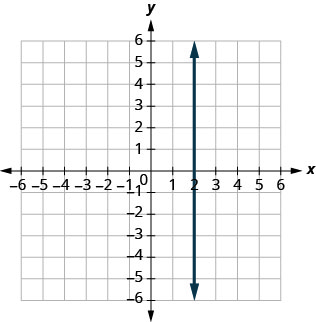
iii. Observe the slope of each line shown.

ⓐ

ⓑ

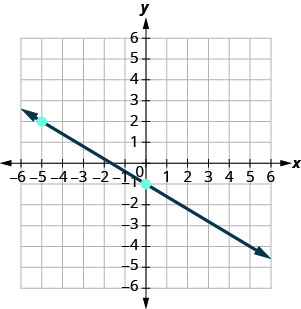
Answer

ⓐ \(−\frac{3}{5}\) ⓑ undefined

iv. Notice the slope of the line between the points \((5,2)\) and \((−1,−4)\).

5. Graph the line with slope \(\frac{1}{2}\) containing the point \((−3,−four)\).

Answer

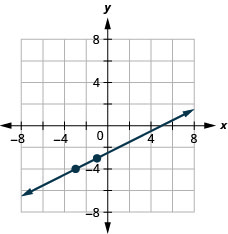
6. Find the intercepts of \(4x+2y=−8\) and graph.

Graph the line for each of the following equations.

7. \(y=\frac{5}{3}x−ane\)

Answer

eight. \(y=−ten\)

nine. \(y=ii\)

Answer

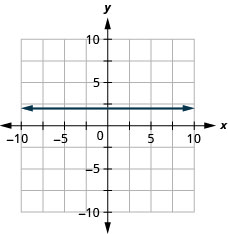
Find the equation of each line. Write the equation in slope-intercept form.

10. slope \(−\frac{3}{4}\) and \(y\)-intercept \((0,−ii)\)

xi. \(chiliad=2\), point \((−three,−1)\)

Reply

\(y=2x+5\)

12. containing \((10,ane)\) and \((6,−1)\)

xiii. perpendicular to the line \(y=\frac{five}{4}x+2\), containing the point \((−10,3)\)

Answer

\(y=−\frac{4}{5}10−5\)

14. Write the inequality shown by the graph with the purlieus line \(y=−x−3\).

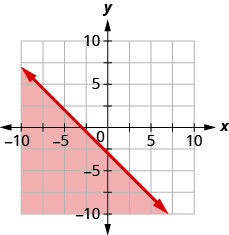
Graph each linear inequality.

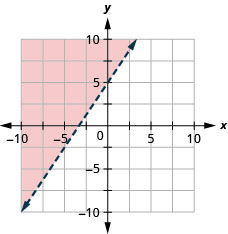
15. \(y>\frac{3}{2}ten+5\)

Answer

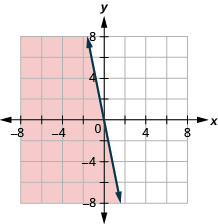
sixteen. \(x−y\geq −4\)

17. \(y\leq −5x\)

Answer

18. Hiro works ii office time jobs in order to earn enough money to meet her obligations of at least $450 a week. Her chore at the mall pays $x an hour and her administrative assistant job on campus pays $xv an hr. How many hours does Hiro need to work at each task to earn at least $450?

ⓐ Permit x exist the number of hours she works at the mall and let y be the number of hours she works every bit administrative banana. Write an inequality that would model this situation.
ⓑ Graph the inequality .
ⓒ Find three ordered pairs \((10,y)\) that would be solutions to the inequality. Then explain what that means for Hiro.

19. Use the set of ordered pairs to ⓐ determine whether the relation is a part, ⓑ find the domain of the relation, and ⓒnotice the range of the relation.

\({\{(−3,27),(−2,8),(−ane,one),(0,0),
(i,1),(2,8),(3,27)}\}\)

Answer

ⓐ yes ⓑ \({\{−three,−2,−1,0,1,2,three}\}\) ⓒ \({\{0, 1, eight, 27}\}\)

twenty. Evaluate the role: ⓐ \(f(−i)\) ⓑ \(f(2)\) ⓒ \(f(c)\).

\(f(ten)=4x^2−2x−3\)

22. Determine whether the graph is the graph of a function. Explain your answer.

In the following exercises, ⓐ graph each function ⓑ state its domain and range.
Write the domain and range in interval notation.

23. \(f(x)=x^two+1\)

Answer

ⓐ

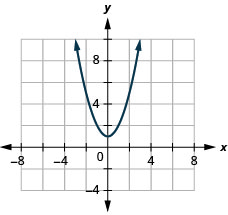
ⓑ \(D: (-\inf ,\inf ), R: [1,\inf )\)

24. \(f(10)=\sqrt{x+one}\)

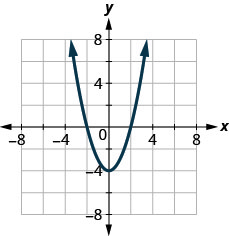
ⓑ Find the \(y\)-intercepts.
ⓒ Find \(f(−i)\).
ⓓ Find \(f(1)\).
ⓔ Discover the domain. Write information technology in interval notation.
ⓕ Find the range. Write it in interval annotation.

Answer

ⓐ \(x=−2,2\) ⓑ \(y=−4\)
ⓒ \(f(−1)=−3\) ⓓ \(f(1)=−iii\)
ⓔ \(D: (-\inf ,\inf )\) ⓕ \(R: [−four, \inf)\)

clarkthled1945.blogspot.com

Source: https://math.libretexts.org/Bookshelves/Algebra/Intermediate_Algebra_%28OpenStax%29/03:_Graphs_and_Functions/3.0E:_3.E:_Chapter_Exercises

Share this post